The poisson solver example..
This example depends on simple-solver.
Introduction
This example solves a 1D Poisson equation:
![$ u : [0, 1] \rightarrow R\\ u'' = f\\ u(0) = u0\\ u(1) = u1 $](form_131.png)
using a finite difference method on an equidistant grid with K discretization points (K can be controlled with a command line parameter). The discretization is done via the second order Taylor polynomial:
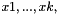
For an equidistant grid with K "inner" discretization points 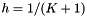 and step size
and step size 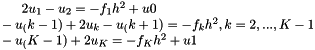 , the formula produces a system of linear equations
, the formula produces a system of linear equations
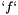
which is then solved using Ginkgo's implementation of the CG method preconditioned with block-Jacobi. It is also possible to specify on which executor Ginkgo will solve the system via the command line. The function 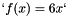 is set to
is set to 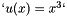 (making the solution
(making the solution 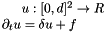 ), but that can be changed in the
), but that can be changed in the main function.
The intention of the example is to show how Ginkgo can be used to build an application solving a real-world problem, which includes a solution of a large, sparse linear system as a component.
About the example
The commented program
row_ptrs[i + 1] = pos;
}
}
Generates the RHS vector given f and the boundary conditions.
template <typename Closure, typename ValueType>
void generate_rhs(Closure f, ValueType u0, ValueType u1,
{
const auto discretization_points =
rhs->get_size()[0];
auto values =
rhs->get_values();
const ValueType h = 1.0 / static_cast<ValueType>(discretization_points + 1);
const auto xi = static_cast<ValueType>(i + 1) * h;
values[i] = -f(xi) * h * h;
}
values[0] += u0;
values[discretization_points - 1] += u1;
}
Prints the solution u.
template <typename Closure, typename ValueType>
void print_solution(ValueType u0, ValueType u1,
{
std::cout << u0 << '\n';
for (
int i = 0; i < u->
get_size()[0]; ++i) {
}
std::cout << u1 << std::endl;
}
Computes the 1-norm of the error given the computed u and the correct solution function correct_u.
template <typename Closure, typename ValueType>
Closure correct_u)
{
const ValueType h = 1.0 / static_cast<ValueType>(discretization_points + 1);
for (int i = 0; i < discretization_points; ++i) {
using std::abs;
const auto xi = static_cast<ValueType>(i + 1) * h;
error +=
}
return error;
}
int main(int argc, char* argv[])
{
Some shortcuts
using ValueType = double;
using IndexType = int;
Print version information
if (argc == 2 && (std::string(argv[1]) == "--help")) {
std::cerr << "Usage: " << argv[0]
<< " [executor] [DISCRETIZATION_POINTS]" << std::endl;
std::exit(-1);
}
Get number of discretization points
const unsigned int discretization_points =
argc >= 3 ? std::atoi(argv[2]) : 100;
Get the executor string
const auto executor_string = argc >= 2 ? argv[1] : "reference";
Figure out where to run the code
std::map<std::string, std::function<std::shared_ptr<gko::Executor>()>>
exec_map{
{"cuda",
[] {
}},
{"hip",
[] {
}},
{"dpcpp",
[] {
}},
{"reference", [] { return gko::ReferenceExecutor::create(); }}};
executor where Ginkgo will perform the computation
const auto exec = exec_map.at(executor_string)();
executor used by the application
Set up the problem: define the exact solution, the right hand side and the Dirichlet boundary condition.
auto correct_u = [](ValueType x) { return x * x * x; };
auto f = [](ValueType x) { return ValueType(6) * x; };
auto u0 = correct_u(0);
auto u1 = correct_u(1);
initialize matrix and vectors
auto matrix = mtx::create(app_exec,
gko::dim<2>(discretization_points),
3 * discretization_points - 2);
generate_stencil_matrix(matrix.get());
auto rhs = vec::create(app_exec,
gko::dim<2>(discretization_points, 1));
generate_rhs(f, u0, u1,
rhs.get());
auto u = vec::create(app_exec,
gko::dim<2>(discretization_points, 1));
for (int i = 0; i < u->get_size()[0]; ++i) {
u->get_values()[i] = 0.0;
}
Generate solver and solve the system
cg::build()
.with_criteria(
gko::stop::Iteration::build().with_max_iters(discretization_points),
reduction_factor))
.with_preconditioner(bj::build())
.on(exec)
->generate(
clone(exec, matrix))
->apply(rhs, u);
Uncomment to print the solution print_solution<ValueType>(u0, u1, u.get());
std::cout << "Solve complete.\nThe average relative error is "
<< calculate_error(discretization_points, u.get(), correct_u) /
discretization_points)
<< std::endl;
}
Results
This is the expected output:
Solve complete.
The average relative error is 2.52236e-11
Comments about programming and debugging
The plain program
#include <iostream>
#include <map>
#include <string>
#include <vector>
#include <ginkgo/ginkgo.hpp>
template <typename ValueType, typename IndexType>
{
const auto discretization_points = matrix->
get_size()[0];
int pos = 0;
const ValueType coefs[] = {-1, 2, -1};
row_ptrs[0] = pos;
for (int i = 0; i < discretization_points; ++i) {
for (auto ofs : {-1, 0, 1}) {
if (0 <= i + ofs && i + ofs < discretization_points) {
values[pos] = coefs[ofs + 1];
col_idxs[pos] = i + ofs;
++pos;
}
}
row_ptrs[i + 1] = pos;
}
}
template <typename Closure, typename ValueType>
void generate_rhs(Closure f, ValueType u0, ValueType u1,
{
const auto discretization_points =
rhs->get_size()[0];
auto values =
rhs->get_values();
const ValueType h = 1.0 / static_cast<ValueType>(discretization_points + 1);
const auto xi = static_cast<ValueType>(i + 1) * h;
values[i] = -f(xi) * h * h;
}
values[0] += u0;
values[discretization_points - 1] += u1;
}
template <typename Closure, typename ValueType>
void print_solution(ValueType u0, ValueType u1,
{
std::cout << u0 << '\n';
for (
int i = 0; i < u->
get_size()[0]; ++i) {
}
std::cout << u1 << std::endl;
}
template <typename Closure, typename ValueType>
Closure correct_u)
{
const ValueType h = 1.0 / static_cast<ValueType>(discretization_points + 1);
for (int i = 0; i < discretization_points; ++i) {
using std::abs;
const auto xi = static_cast<ValueType>(i + 1) * h;
error +=
}
return error;
}
int main(int argc, char* argv[])
{
using ValueType = double;
using IndexType = int;
if (argc == 2 && (std::string(argv[1]) == "--help")) {
std::cerr << "Usage: " << argv[0]
<< " [executor] [DISCRETIZATION_POINTS]" << std::endl;
std::exit(-1);
}
const unsigned int discretization_points =
argc >= 3 ? std::atoi(argv[2]) : 100;
const auto executor_string = argc >= 2 ? argv[1] : "reference";
std::map<std::string, std::function<std::shared_ptr<gko::Executor>()>>
exec_map{
{"cuda",
[] {
}},
{"hip",
[] {
}},
{"dpcpp",
[] {
}},
{"reference", [] { return gko::ReferenceExecutor::create(); }}};
const auto exec = exec_map.at(executor_string)();
auto correct_u = [](ValueType x) { return x * x * x; };
auto f = [](ValueType x) { return ValueType(6) * x; };
auto u0 = correct_u(0);
auto u1 = correct_u(1);
auto matrix = mtx::create(app_exec,
gko::dim<2>(discretization_points),
3 * discretization_points - 2);
generate_stencil_matrix(matrix.get());
auto rhs = vec::create(app_exec,
gko::dim<2>(discretization_points, 1));
generate_rhs(f, u0, u1,
rhs.get());
auto u = vec::create(app_exec,
gko::dim<2>(discretization_points, 1));
for (int i = 0; i < u->get_size()[0]; ++i) {
u->get_values()[i] = 0.0;
}
cg::build()
.with_criteria(
gko::stop::Iteration::build().with_max_iters(discretization_points),
reduction_factor))
.with_preconditioner(bj::build())
.on(exec)
->generate(
clone(exec, matrix))
->apply(rhs, u);
std::cout << "Solve complete.\nThe average relative error is "
<< calculate_error(discretization_points, u.get(), correct_u) /
discretization_points)
<< std::endl;
}


![$ u : [0, 1] \rightarrow R\\ u'' = f\\ u(0) = u0\\ u(1) = u1 $](form_131.png)
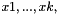
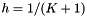 and step size
and step size 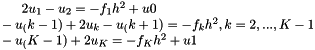 , the formula produces a system of linear equations
, the formula produces a system of linear equations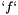
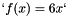 is set to
is set to 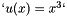 (making the solution
(making the solution 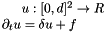 ), but that can be changed in the
), but that can be changed in the  1.8.16
1.8.16